Professor Tony Padilla on the epic number, TREE(3). Continues at: https://youtu.be/IihcNa9YAPk
Some math conjectures and theorems and proofs can take on a profound, quasi-religious status as examples of the limits of human comprehension. TREE(3) is one of those examples.
“You’ve got all these physical processes going on in the universe all around you. None of them are anything compared to TREE(3),” says University of Nottingham mathmatics professor Tony Padilla in a new episode of the wonderful YouTube series Numberphile.
What is TREE(3)? It’s a number. An enormous number beyond our ability to express with written notation, beyond what we could even begin to comprehend, bigger than the notoriously gargantuan Graham’s number. We know TREE(3) exists, and we know it’s finite, but we do not know what it is or even how many digits there are.
The number comes from a simple game of trees—meaning the charts used in graph theory. In this game, you make a forest of trees using seeds. In other words, you make as many tree graphs as you can with a combination of different colored units referred to as “seeds.”
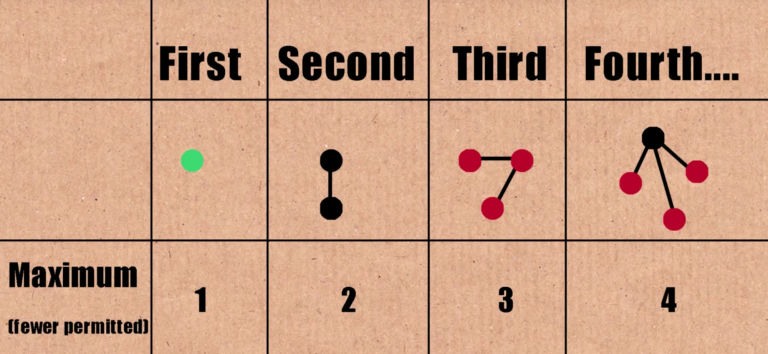
There are only two rules. The first rule is that the first tree must contain no more than one seed, the second a maximum of two seeds, the third a maximum of three, and so on. It will look something like this:
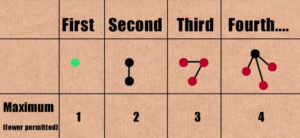
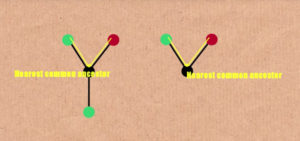
The second rule is this: When you make a tree that a previous tree could be contained within, the game end.d. Ss Padilla says, “the forest dies.” What exactly does this mean? To put it another way: If you make a tree graph that contains a previous smaller tree graph, the game ends. A tree is said to be contained within another tree if it has seeds at the ends that share a common seed earlier in the graph, or common ancestor, and that pattern is also present in the larger tree graph. The video above will give you a much better sense with examples, but it looks something like this:
The point of this game is to make as many trees as you can before you inevitably make one that contains a previous tree, and the forest dies and the game ends. To start, just use one type or color of seed, which is TREE(1). Following the two rules to the game, you can quickly see that after the first tree (which is only one seed), a second tree is built that contains the first, and the game ends after just one step. So TREE(1) = 1.
More Info:
Graham’s Number: http://bit.ly/G_Number
Numberphile is supported by the Mathematical Sciences Research Institute (MSRI): http://bit.ly/MSRINumberphile
We are also supported by Science Sandbox, a Simons Foundation initiative dedicated to engaging everyone with the process of science.
Partial Source: http://www.popularmechanics.com/science/math/a28725/number-tree3/