Tag: Trichotomy

9. Triadism and the Universal Categories

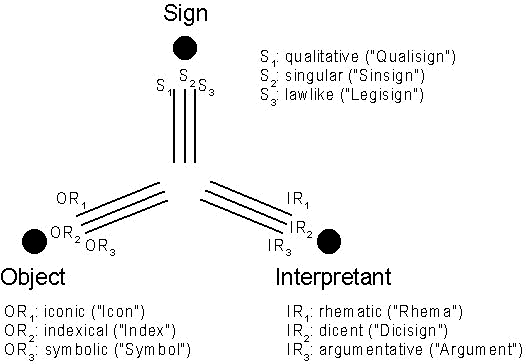
Merely to say that Peirce was extremely fond of placing things into groups of three, of trichotomies, and of triadic relations, would fail miserably to do justice to the overwhelming obtrusiveness in his philosophy of the number three.

Indeed, he made the most fundamental categories of all “things” of any sort whatsoever the categories of “Firstness,” “Secondness,” and “Thirdness,” and he often described “things” as being “firsts” or “seconds” or “thirds.”

For example, with regard to the trichotomy “possibility,” “actuality,” and “necessity,” possibility he called a first, actuality he called a second, and necessity he called a third. Again: quality was a first, fact was a second, and habit (or rule or law) was a third. Again: entity was a first, relation was a second, and representation was a third. Again: rheme (by which Peirce meant a relation of arbitrary adicity or arity) was a first, proposition was a second, and argument was a third.

The list goes on and on. Let us refer to Peirce’s penchant for describing things in terms of trichotomies and triadic relations as Peirce’s “triadism.”

Continue reading C. S. Peirce – Triadism and the Universal Categories