triangulation

Posted on October 22, 2022October 22, 2022 by 3

Pythagorean triple

A Pythagorean triple consists of three positive integers a, b, and c, such that a² + b² = c². Such a triple is commonly written (a, b, c), and a well-known example is (3, 4, 5). If (a, b, c) is a Pythagorean triple, then so is (ka, kb, kc) for any positive integer k. A primitive Pythagorean triple is one in which a, b and c are coprime (that is, they have no common divisor larger than 1). For example, (3, 4, 5) is a primitive Pythagorean triple whereas (6, 8, 10) is not. A triangle whose sides form a Pythagorean triple is called a Pythagorean triangle, and is necessarily a right triangle.

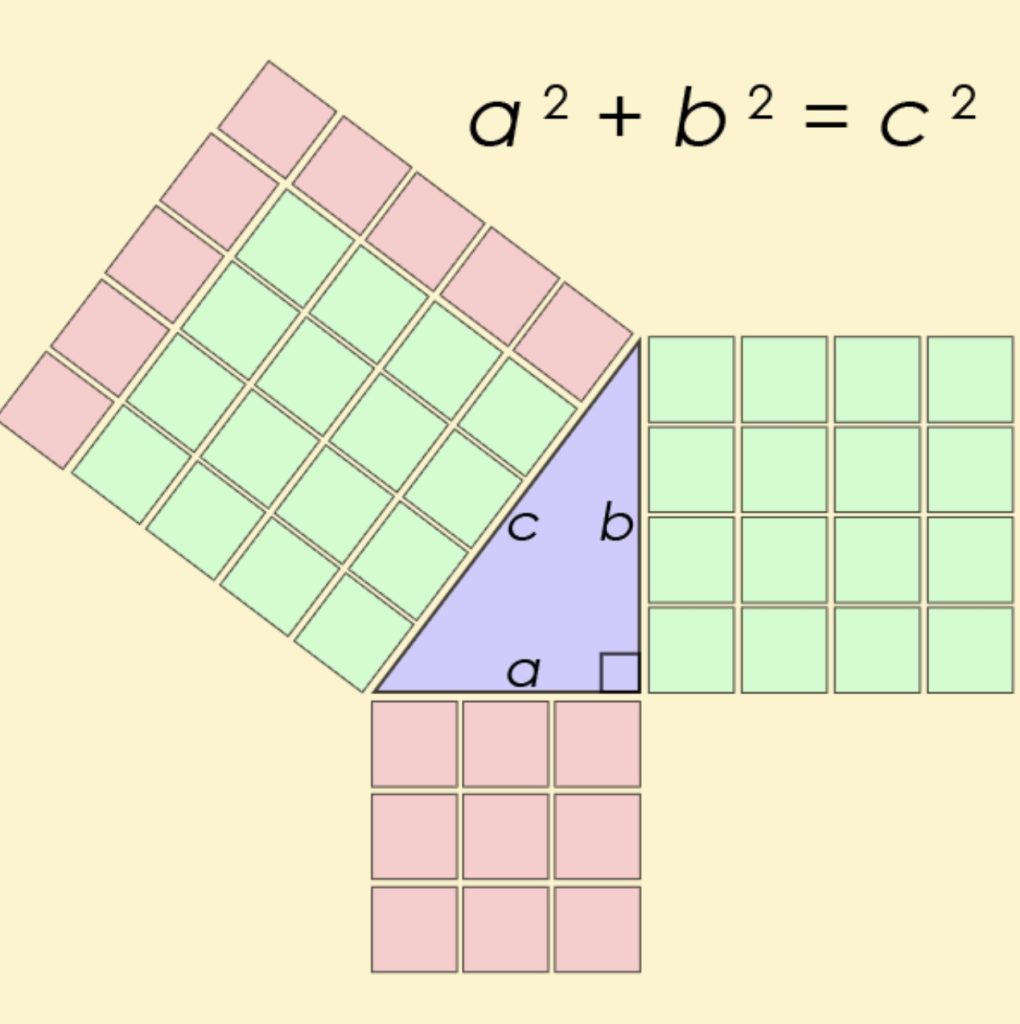
The name is derived from the Pythagorean theorem, stating that every right triangle has side lengths satisfying the formula a² + b² = c²; thus, Pythagorean triples describe the three integer side lengths of a right triangle. However, right triangles with non-integer sides do not form Pythagorean triples. For instance, the triangle with sides a=b=1 and c=2 is a right triangle, but (1,1,2) is not a Pythagorean triple because 2 is not an integer. Moreover, 1and 2 do not have an integer common multiple because 2 is irrational.

Pythagorean triples have been known since ancient times. The oldest known record comes from Plimpton 322, a Babylonian clay tablet from about 1800 BC, written in a sexagesimal number system. It was discovered by Edgar James Banks shortly after 1900, and sold to George Arthur Plimpton in 1922, for $10.

Plimpton 322, a Babylonian clay tablet from about 1800 BC

One example of a Pythagorean triple is a=3, b=4, and c=5: Ancient Egyptians used this group of Pythagorean triples to measure out right angles. They would tie knots in a piece of rope to create 3, 4, and 5 equal spaces. Three people would then hold each corner of the rope and form a right triangle!

right triangles during the construction process to help determine the slope of the pyramid. The Pythagorean Theorem states that given a right triangle with sides of length a and b respectively and a hypothenuse of length c, the lengths satisfy the equation a² + b² = c^2.

Pyramids at Giza — All three of Giza’s famed pyramids and their elaborate burial complexes were built during a frenetic period of construction, from roughly 2550 to 2490 B.C.

When searching for integer solutions, the equation a² + b² = c² is a Diophantine equation. Thus Pythagorean triples are among the oldest known solutions of a nonlinear Diophantine equation. The simplest linear Diophantine equation takes the form ax + by = c, where a, b and c are given integers. The solutions are described by the following theorem: This Diophantine equation has a solution (where x and y are integers) if and only if c is a multiple of the greatest common divisor of a and b.

Posted on June 27, 2022July 14, 2022 by 3

The Metre – the repeating circle & triangulation

The Metre (meaning measure) was one ten-millionth of the distance from the North Pole to the Equator! France embarked on a first large scale measurement. It took 7 years to measure the distance from Dunkirk to Barsalona. They used triangulation with an instrument called the Repeating Circle along with trigonometry.
The standardization of measurement: the Metre

Creating the Metre – a universal standard

By the 16th century, there we over 250,000 weights and measures in Europe. This effected trade, navigation, building plans, etc. Fire hoses would not connect from town to town. France chose to create a standard by measuring something unchangeable. They chose the Earth. Before this standardization, the human body (the Ruler of the land) would make new measurements upon gaining power.

The Repeating Circle

DESCRIPTION

This is one of two double repeating circles that Ferdinand Rudolph Hassler, the first superintendent of the U. S. Coast Survey, ordered from Edward Troughton in London in 1812, and that was shipped in 1815. The large circle may be angled from vertical to horizontal to the opposite vertical position. It is graduated to 10 minutes, and read by four verniers and two magnifiers to single minutes.

A repeating circle is a geodetic instrument with two telescopes that is designed to reduce errors by repeated observations taken on all parts of the circumference of a circle. The form was developed by the Chevalier de Borda, first executed by Etienne Lenoir in Paris around 1789, and popular for about 50 years.

Ref: F. R. Hassler, “Papers on Various Subjects Connected with the Survey of the Coast of the United States,” Transactions of the American Philosophical Society 2 (1825): 232-420, on 315-320 and pl. VII. “The Repeating Circle Without Reflection, as made by Troughton,” in The Cyclopaedia: or, Universal Dictionary of Arts, Sciences, and Literature, edited by Abraham Rees (London, 1819), Vol. VII, Art “Circle.”

Image credit:

NAME: repeating circle MAKER: Troughton and Simms PLACE MADE: United Kingdom: England, London MEASUREMENTS: overall: 32 1/8 in x 26 3/4 in x 17 in; 81.6356 cm x 67.945 cm x 43.18 cm upper circle: 17 1/2 in; 44.45 cm circle at base: 13 1/2 in; 34.29 cm telescope: 24 in; 60.96 cm overall; base: 16 3/4 in x 15 1/4 in x 16 in; 42.545 cm x 38.735 cm x 40.64 cm overall; horizontal circle: 13 in x 23 in x 20 in; 33.02 cm x 58.42 cm x 50.8 cm ID NUMBER PH.314640 CATALOG NUMBER 314640 ACCESSION NUMBER 208213

Posted on January 26, 2011March 9, 2014 by 3 — Leave a comment

Triangulation

The name given to the act of a political candidate presenting his or her ideology as being “above” and “between” the “left” and “right” sides (or “wings”) of a traditional (e.g. UK or US) democratic “political spectrum”. It involves adopting for oneself some of the ideas of one’s political opponent (or apparent opponent). The logic behind it is that it both takes credit for the opponent’s ideas, and insulates the triangulator from attacks on that particular issue. Opponents of triangulation, who believe in a fundamental “left” and “right”, consider the dynamic a deviation from its “reality” and dismiss those that strive for it as whimsical.
Source: Wikipedia

Obama: Triangulation 2.0?
Published on Monday, January 24, 2011 by The Nation by Ari Berman

Immediately following the Democrats’ 2010 electoral shellacking, a broad spectrum of pundits urged President Obama to “pull a Clinton,” in the words of Politico: move to the center (as if he wasn’t already there), find common ground with the GOP and adopt the “triangulation” strategy employed by Bill Clinton after the Democratic setback in the 1994 midterms. “Is ‘triangulation’ just another word for the politics of the possible?” asked the New York Times. “Can Obama do a Clinton?” seconded The Economist. And so on. The Obama administration, emphatic in charting its own course, quickly took issue with the comparison. According to the Times, Obama went so far as to ban the word “triangulation” inside the White House. Politico called the phrase “the dirtiest word in politics.”

Continue reading Triangulation