What is the composite function f o g?
f is the outer function; g is the inner function. `Suppose f(x) = x-2 and g(x) = x2. (a) Find fog and gof. (fog)(x) = f(g(x)) = f(x2) = x2-2.
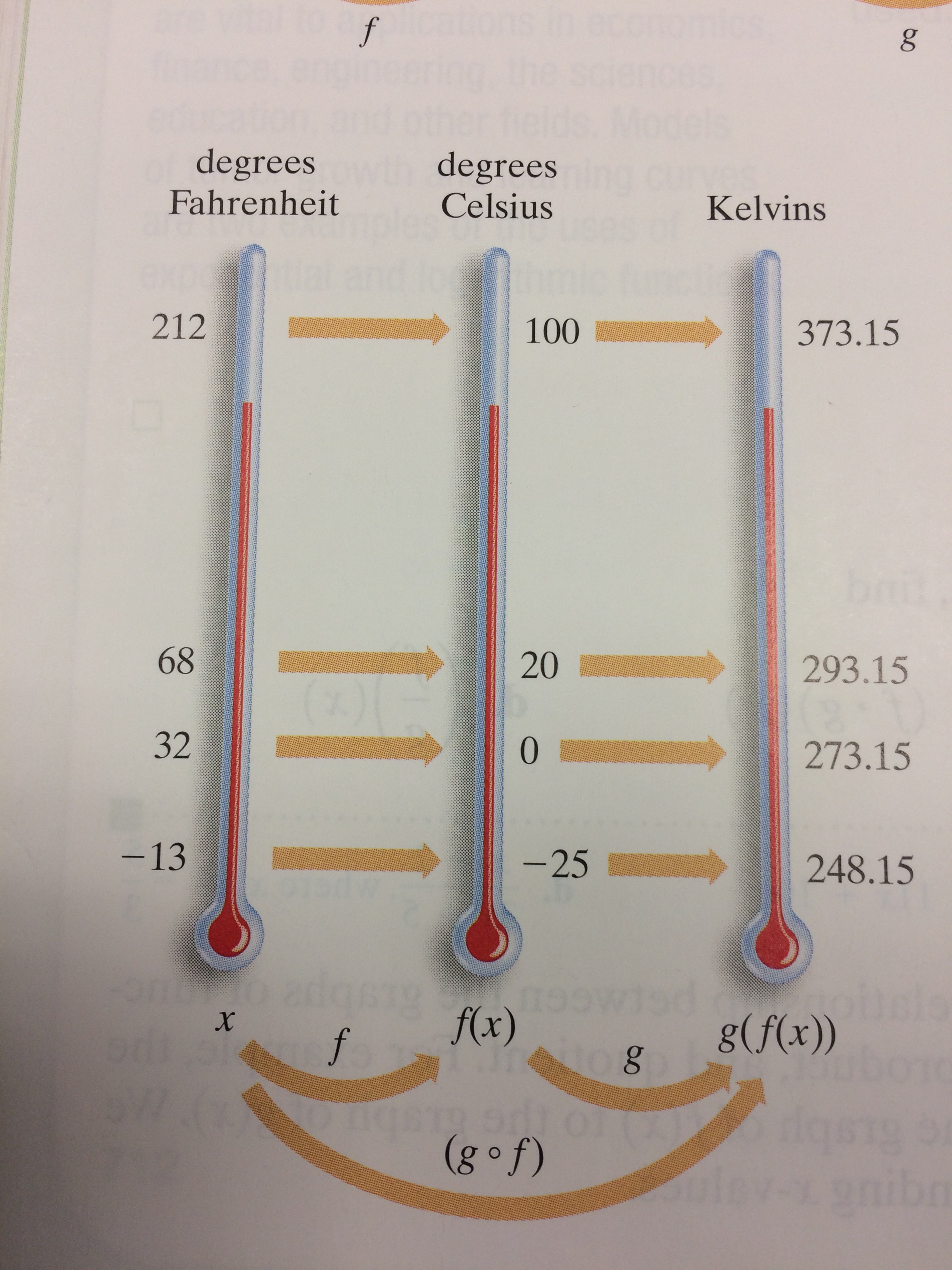
In 1954, the tenth general conference on weights and measures adopted the Kelvin K as the basic unit for measuring all international weights and measures. While the kelvin is the standard unit, degrees Fahrenheit and degrees Celsius are still in common use in the United States.

The function C(F)=5/9(F-32) relates Celsius temperatures and Fahrenheit Temperatures. The function K(C)= C + 273.15 relates celsius temperatures and kelvin temperatures.
Let’s convert Fahrenheit to Kelvin. The composition of the function K with the function C is
K(C(F)) = C(F) + 273 = (5/9)(F-32) + 273
Since C converts Fahrenheit to Celsius and K converts Celsius to Kelvin, the composition will convert Fahrenheit to Kelvin.
In English:
- To convert Fahrenheit to Celsius, subtract 32 from the Fahrenheit temperature and then divide your answer by 1.8.
- To convert from Celsius to Fahrenheit, multiply the Celsius temperature by 1.8 and then add 32 to your answer.
- If you’re trying to convert Celsius to Kelvin, just add 273.15 to the Celsius temperature.
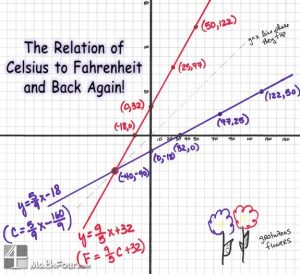
At what temperature are Fahrenheit and Celsius the same?
Let’s solve a system of linear equations graphically then algebraically.
Graphing solution:
Algebraic solution:
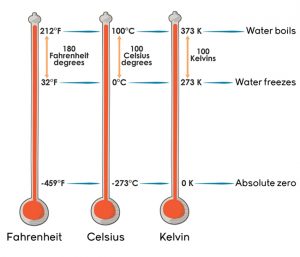
The formulas for converting between degree Celsius and degree Fahrenheit are:
°F = (°C * 9/5) + 32
°C = (°F – 32) * 5/9
To find the temperature when both are equal, we use an old algebra trick and just set ºF = ºC and solve one of the equations.
°C = (°C * 9/5) + 32
°C – (°C * 9/5) = 32
-4/5 * °C = 32
°C = -32 * 5/4
°C = -40
°F = (°F * 9/5) + 32
°F – (°F * 9/5) = 32
-4/5 * °F = 32
°F = -32 * 5/4
°F = -40
So the temperature when both the Celsius and Fahrenheit scales are the same is -40 degrees.